How To Find Sampling Distribution Of Sample Mean
Probability and Statistics > Sampling > Sampling Distribution
Contents (click to skip to that section):
- What is a Sampling Distribution?
- Mean of the sampling distribution of the mean
- Hateful of Sampling Distribution of the Proportion
- Standard Divergence of Sampling Distribution of the Proportion
Definition
Picket the video for an overview:
What is a Sampling Distribution?
Can't see the video? Click here.
A sampling distribution is a graph of a statistic for your sample data. While, technically, yous could choose whatever statistic to paint a motion picture, some mutual ones y'all'll come beyond are:
- Mean
- Hateful accented value of the departure from the mean
- Range
- Standard deviation of the sample
- Unbiased guess of variance
- Variance of the sample
Upwardly until this point in statistics, you've probably been plotting graphs for a gear up of numbers. For case, you might have graphed a data set and found it follows the shape of a normal distribution with a mean score of 100. Where probability distributions differ is that you aren't working with a single set up of numbers; y'all're dealing with multiple statistics for multiple sets of numbers. If you find that concept hard to grasp: you aren't lone.
While most people can imagine what the graph of a set of numbers looks like, it's much more than difficult to imagine what stacks of, say, averages look like.
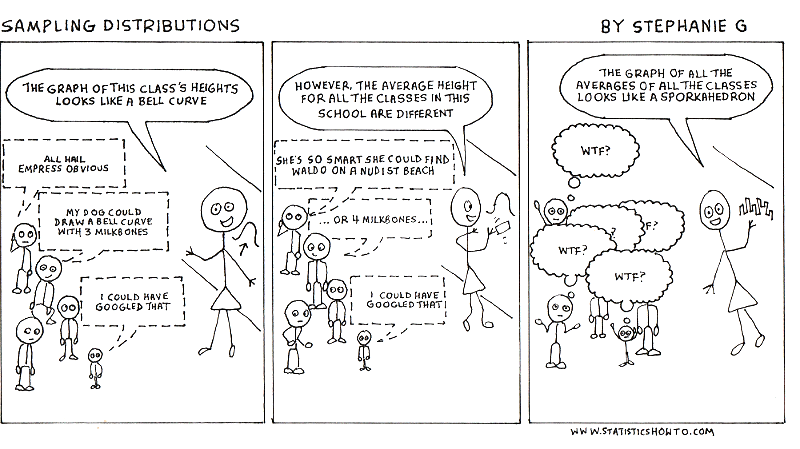
An explanation…
Let's get-go with a mean, like heights of students in the higher up cartoon. As y'all probably know, heights (and many other natural phenomenon) follow a bell curve shape. So if you surveyed your course, you lot'd probably find a few short people, a few tall people, and virtually people would fall in betwixt. Let's say the average height was 5'9″. Survey all the classes in your school and you'll probably get somewhere shut to the boilerplate. If you had 10 classes of students, you might become 5'nine″, 5'8″, five'10", 5'9″, 5'7″, v'ix″, 5'nine″, five'ten", v'7″, and v'9″. If you graph all of those averages, you're probably going to get a graph that resembles the "sporkahedron." For other information sets, you might get something that looks flatlined, like a uniform distribution. It's almost impossible to predict what that graph will look similar, but the Primal Limit Theorem tells us that if you accept a ton of data, it'll eventually look like a bell curve. That'southward the basic idea: you lot have your average (or another statistic, like the variance) and you plot those statistics on a graph.
This video introduces the Cardinal Limit Theorem every bit information technology applies to these distributions. The "mean of the sampling distribution of the ways" is but math-speak for plotting a graph of averages (like I outlined above) and then finding the boilerplate of that set of data.
mean of the sampling distribution of the hateful
Back to Top
Mean of the sampling distribution of the mean
In a nutshell, this is the aforementioned as the population mean. For example, if your population mean (μ) is 99, then the hateful of the sampling distribution of the hateful, μm, is also 99 (as long as you have a sufficiently large sample size). If you want to understand why, watch the video or read on below.
mean of the sampling distribution of the mean
The Central Limit Theorem.
Roughly stated, the central limit theorem tells u.s.a. that if we have a large number of independent, identically distributed variables, the distribution will approximately follow a normal distribution. Information technology doesn't matter what the underlying distribution is.
Here's a uncomplicated case of the theory: when you roll a single die, your odds of getting whatever number (one,2,3,4,5, or 6) are the aforementioned (1/half-dozen). The mean for whatsoever roll is (1 + 2 + 3 + iv + 5 + 6) / 6 = 3.5. The results from a one-die roll are shown in the first figure below: it looks like a uniform distribution. Notwithstanding, as the sample size is increased (two dice, 3 dice…), the distribution of the mean looks more and more like a normal distribution. That is what the central limit theorem predicts.
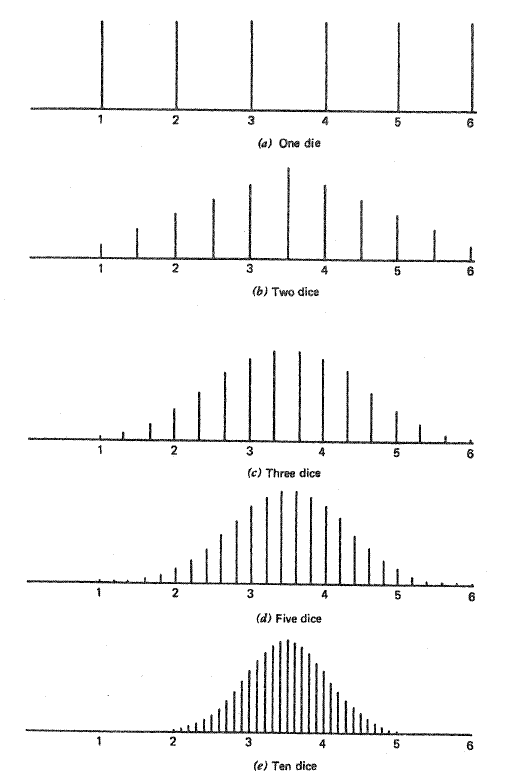
Image: U of Michigan.
Equally the sample size increases, distribution of the mean will approach the population hateful of μ, and the variance volition approach σ2/N, where North is the sample size.
You tin can recollect of a sampling distribution as a relative frequency distribution with a large number of samples.
The hateful of the sampling distribution of the mean formula
The formula is μThou = μ, where μM is the mean of the sampling distribution of the mean.
Mean of Sampling Distribution of the Proportion
The mean of sampling distribution of the proportion, P, is a special instance of the sampling distribution of the mean. The hateful of the sampling distribution of the proportion is related to the binomial distribution.
A Binomial Distribution is related to Mean of Sampling Distribution of the Proportion.
Proportions are something you probably already know. For example: 100 people are asked if they are democrat. If 50 people answer "yes" then the sample proportion p = 50/100. Technically (the "mathy fashion"): A sample proportion is where a random sample of objects n is taken from a population P; if x objects have a sure characteristic and so the sample proportion "p" is: p = ten/n.
The sampling distribution of a proportion is when you repeat your survey or poll for all possible samples of the population. For example: instead of polling asking 1000 cat owners what cat nutrient their pet prefers, you could repeat your poll multiple times.
Mean of Sampling Distribution of the Proportion
If a random sample of due north observations is taken from a binomial population with parameter p, the sampling distribution (i.e. all possible samples taken from the population) will have a hateful up=p. With a large sample, the sampling distribution of a proportion will accept an approximate normal distribution.
Standard Deviation of Sampling Distribution of the Proportion
The standard deviation of sampling distribution of the proportion, P, is also closely related to the binomial distribution and is a special case of a sampling distribution.
Case: Y'all hold a survey about higher student'due south GRE scores and calculate that the standard divergence is 1. It is highly unlikely that yous will get the same results if yous echo the survey (you might get 1.1 ,1.2 or 0.nine). Therefore you'll want to echo the poll the maximum number of times possible (i.e. you describe all possible samples of size n from the population). Y'all'll have a range of standard deviations — one for each sample.
Sampling Distribution of a Proportion
This is when yous repeat your survey for all possible samples of the population. For case: instead of polling 100 people once to enquire if they are democrat, you lot'll poll them multiple times to go a better estimate of your statistic.
Standard Deviation
If a random sample of n observations is taken from a binomial population with parameter p, the sampling distribution (i.east. all possible samples taken from the population) will have a standard departure of:
Standard deviation of binomial distribution = σp = √[pq/n] where q=1-p.
When the sample is large, the distribution will take an gauge normal distribution.
Check out our YouTube channel for more tips and help for stats!
References
Everitt, B. S.; Skrondal, A. (2010), The Cambridge Dictionary of Statistics, Cambridge University Press.
Gonick, L. (1993). The Drawing Guide to Statistics. HarperPerennial.
Kotz, S.; et al., eds. (2006), Encyclopedia of Statistical Sciences, Wiley.
Levine, D. (2014). Even Y'all Tin can Larn Statistics and Analytics: An Easy to Sympathise Guide to Statistics and Analytics third Edition. Pearson FT Press
---------------------------------------------------------------------------
Demand aid with a homework or test question? With Chegg Written report, you tin get step-by-step solutions to your questions from an expert in the field. Your kickoff 30 minutes with a Chegg tutor is costless!
Comments? Need to postal service a correction? Please post a comment on our Facebook folio .
Source: https://www.statisticshowto.com/sampling-distribution/
Posted by: brownspoks1979.blogspot.com


0 Response to "How To Find Sampling Distribution Of Sample Mean"
Post a Comment